In geometry, cone is a solid or hollow object with a round flat base and sides that slope up to a top point. The cone formulas, solved example & step by step calculations may useful for users to understand how the input values are being used in such calculations. Also this featured cone calculator uses the various conversion functions to find its area, volume & slant height in SI or metric or US customary units.
We can also define the cone as a pyramid with a circular cross-section, unlike a pyramid that has a triangular cross-section. Let us study the cone height formula using solved examples at the end of the page. To calculate the total surface area of a cone we need radius of circular base and height of cone. Then, it calculates the total surface area of cone using the formula given above and prints the result on screen using printf function. In the field of geometry calculations, finding the area, volume & slanting height of a cone is very important to understand a part of basic mathematics.
A cone is an three-dimensional geometric shape having circular base and only one vertex. A cone can be formed by the locus of all straight line segments that join the vertex to the base has a rotational symmetry. We can say that a cone have flat circular base and having one side curved surface. The volume and the total surface area of the cone depend upon the radius of base, height and slant height of the cone.
The area occupied by the surface/boundary of a cone is known as the surface area of a cone. Stacking many triangles and rotating them around an axis gives the shape of a cone. As it has a flat base, thus it has a total surface area as well as a curved surface area. We can classify a cone as a right circular cone or an oblique cone.
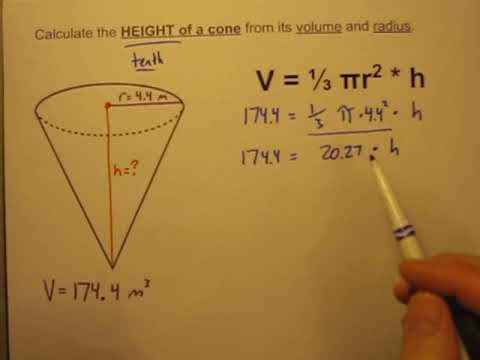
The cone height formula helps in calculating the distance from the vertex of the cone to the cone's base. The height of the cone can be calculated using either the volume of cube and radius or with slant height and radius of the cone. The volume of a cone can be defined as the amount of three dimensional space occupied by the right circular cone or the storage capacity of a right circular cone. Finding volume of a cone help us to solve many real life problems like, how much ice-cream is required to file an ice-cream cone. To calculate the volume of a right circular cone, we need radius of base height of cone. Volume of a cone is measured in cubic units like meter3, cm3 etc.
Let's get right to it — we're here to calculate the surface area or volume of a right circular cone. As you might already know, in a right circular cone, the height goes from the cone's vertex through the center of the circular base to form a right angle. Right circular cones are what we typically think of when we think of cones. The area of the curved surface of a cone is known as the curved surface area of the cone. The formula to calculate the curved surface area of a cone is πrl where "r" is the radius of the base and "l" is the slant height of the cone.
In this, we do not consider the area of the base of the cone which is the shape of a circle. The surface area of a cone is the amount of area occupied by the surface of a cone. A cone is a 3-D shape that has a circular base. This means the base is made up of a radius or diameter.
The distance between the center of the base and the topmost part of the cone is the height of the cone. Like all three-dimensional shapes, you will learn how to calculate the surface area of a cone in this article. If the cone is right circular the intersection of a plane with the lateral surface is a conic section. In general, however, the base may be any shape and the apex may lie anywhere . Contrasted with right cones are oblique cones, in which the axis passes through the centre of the base non-perpendicularly. Conical and pyramidal shapes are often used, generally in a truncated form, to store grain and other commodities.
Similarly a silo in the form of a cylinder, sometimes with a cone on the bottom, is often used as a place of storage. It is important to be able to calculate the volume and surface area of these solids. A cone is a three dimensional geometric shape with one vertex and a circular base. The line form the centre of the base to the apex is the perpendicular height. Surface area of a cone is the complete area covered by its two surfaces, i.e., circular base area and lateral surface area.
The circular base area can be calculated using area of circle formula. The lateral surface area is the side-area of the cone. Let us see the formula to calculate the surface area of cone. A right circular cone is defined as the cone whose axis is the line joining the vertex and the midpoint of the circular base. Thus, the curved surface area of a right circular cone is given as πrl where "r" is the radius of the base and "l" is the slant height.
In terms of height, the curved surface area of a right circular cone is given as πr(√(h2 + r2)) where "h" is the height of the right circular cone. A cone has a three-dimensional shape so calculating its volume can seem a little complicated. To help you understand better, in this article we explain what a cone is as well as how to calculate its volume. We detail the steps one by one and the formulas you have to use to calculate the volume of a cone with accurate examples.
In this module, we will examine how to find the surface area of a cylinder and develop the formulae for the volume and surface area of a pyramid, a cone and a sphere. These solids differ from prisms in that they do not have uniform cross sections. Cones have a circular base and resemble pyramids. It's a three-dimensional geometry with a smooth base that tapers to a point at the top called the vertex.
We analyze a right circular cone with a circular base when studying how to find the surface area of cones. A cone can be thought of as a triangle rotated around one of its vertices. To calculate the slant height of either a cone or a pyramid, you need to imagine that you can look inside of the figure.
First, we cut down through the cone from vertex point A to segment BC to get two halves. The cut surface of either half is now in the shape of an isosceles triangle, which is a triangle with two sides that are the same length. Those two sides were the slant height of the cone. We now have triangle ABC, where sides AB and AC have the same length.
The slant height of a cone should not be confused with the height of a cone. Slant height is the distance from the top of a cone, down the side to the edge of the circular base. Slant height is calculated as \(\sqrt\), where \(r\) represents the radius of the circular base, and \(h\) represents the height, or altitude, of the cone. A cone folded flat forms a sector of a larger circle.Imagine a cone without its base, made out of paper.
You then roll it out so it lies flat on a table. You will get a shape like the one in the diagram above. It is a part of a larger circle, whose radius is equal to the slant height of the cone. The arc length of the sector is equivalent to the circumference of the cone base. It has a curved surface which tapers (i.e. decreases in size) to a vertex at the top.
The height of the cone is the perpendicular distance from the base to the vertex. Since the flat surface of a cone is circular, the formula for the flat surface of the cone is πr2 square units. It is also called the base surface area of a cone. If you further cut this figure into multiple pieces' viz. Ob1, Ob2, Ob3, …….., Obn each measuring the same length as the slant height of the original cone, you will observe n triangles are formed out of it. Our traffic cone is a little different from the geometric shape called a cone.
In geometry, the base of a cone is only a circle that does not extend beyond the opening of the cone. The point of a cone in geometry is called the vertex point. The slant height and the altitude always meet at that vertex point in a cone. On the traffic cone, the two segments did not meet because the tip is flat and does not come to one point. Identify the radius of the cone's base circle. If you have the diameter, cut it in half to get the radius.
If you have the slant height and perpendicular height, use the Pythagorean theorem. If the enclosed points are included in the base, the cone is a solid object; otherwise it is a two-dimensional object in three-dimensional space. The axis of the cone is the segment whose endpoints are center of the base to vertex or apex.
If the axis of the cone is perpendicular to the plane of the circle, then it is called as a right circular cone otherwise it is an oblique cone. In the video lesson, we learned how to find the slant height of a cone or pyramid when we know the altitude and information about the base. The same formula for slant height can be manipulated to find the altitude, the radius of the base , or half the side length of the base .
The distance along the outside of a cone, from the top to the base, is known as the slant height. The "height" of a cone, and the "slant height" of a cone are not the same thing. The height of a cone is considered the vertical height or altitude of the cone. This is the perpendicular distance from the top of the cone down to the center of the circular base. The slant height of a cone is the distance from the top of the cone, down the side of the cone to the edge of the circular base. The surface area of a cone is the sum of the lateral surface area and the base surface area.
If you know the radius of the base and the slant height of the cone, you can easily find the total surface area using a standard formula. Sometimes, however, you might have the radius and some other measurement, such as the height or volume of the cone. In these instances, you can use the Pythagorean Theorem and the volume formula to derive the slant height, and thus the surface area of the cone. Given slant height, height and radius of a cone, we have to calculate the volume and surface area of the cone. Cone is another important figure in geometry.
Let us take a cone of height "h", base radius "r", and slant height "l". In order to determine the surface area of cone derivation, we cut the cone open from the center which looks like a sector of a circle . The cone height formula using slant height is √l2- r2, where l is the slant height and r is the radius of the cone. This formula is derived using the Pythagoras theorem. A solid metallic cuboid 24cm X 11cm X 7cm is melted and recast into solid cones of base radius 3.5 cm and height 6 cm. The Pythagorean Theorem will be used to calculate the slant height using the radius and height of the cone as the right triangle's legs.
If the circumference of the base of the solid right circular cone is 236 and its slant height is 12 cm, find its curved surface area. In projective geometry, a cylinder is simply a cone whose apex is at infinity. This is useful in the definition of degenerate conics, which require considering the cylindrical conics. The "base radius" of a circular cone is the radius of its base; often this is simply called the radius of the cone. The aperture of a right circular cone is the maximum angle between two generatrix lines; if the generatrix makes an angle θ to the axis, the aperture is 2θ.
The following mathematical formulas are used in this cone calculator to find the area, volume & slanting height of a cone. The radius of base of a right circular cone is 7 cm and its slant height is 25 cm. For an oblique cone, slant height is undefined so there are no formulas for the areas of oblique cones.
A cone is a three-dimensional shape in geometry. The object have curved surface with a vertex and a circular base is called circular cone. But the trick is to figure out how to design a 2-D net for the cone.
Did you know that the 2-D net for a cone is a sector of a circle? Here, the circle we are talking about has radius s . So we know the radius of the sector is s, not r. But the big question is, how big is the angle of the sector?
That is, what is angle A in the figure below? The amount of the circumference of the sector is the same as the whole circumference of the cone's base, namely, 2𝜋r. The total surface area of cone is the total area on the outer side of a cuboid. In other words, It is the number of square units that will exactly cover the outer surface of a cone. A cone is a combination of two surfaces, the curved top section with slanted height and the circular base. Hence, The total surface area of cone is sum of area of circular base and area of top curved section.
Q.1. Determine the curved surface area of a cone whose base radius is 7 cm and slant height is 15 cm. Surface area of a cone is the total area covered by its surface. The total surface area will cover the base area and lateral surface area of the cone.
Cone is defined as a three-dimensional solid structure that has a circular base. A cone can be viewed as a set of non-congruent circular disks that are placed over one another such that the ratio of the radius of adjacent disks remains constant. Figures such as cones and pyramids have two measurements that indicate how tall the figure is.